Sign Up to our MetaHub questions and Answers Engine to ask questions, answer members' questions, and connect with other teachers & members.
Login to our META-HUB questions & Answers Forum
Lost your password? Please enter your email address. You will receive a link and will create a new password via email.
Please briefly explain why you feel this question should be reported.
Please briefly explain why you feel this answer should be reported.
Please briefly explain why you feel this user should be reported.
√ab=√a√b ?
In most of the books that I have read, they simply stressed that √ab=√a√b is valid only when, atleast one of a and b is non-negative. And just giving numerical examples to convince that the validity of √ab=√a√b.(Even in the JEE textbooks). But it works when a = b = i. This confused me a bit and I seRead more
In most of the books that I have read, they simply stressed that √ab=√a√b is valid only when, atleast one of a and b is non-negative. And just giving numerical examples to convince that the validity of √ab=√a√b.(Even in the JEE textbooks). But it works when a = b = i. This confused me a bit and I searched all over the internet but couldn’t find a clear answer.
Thank you very much for the clear explanation!
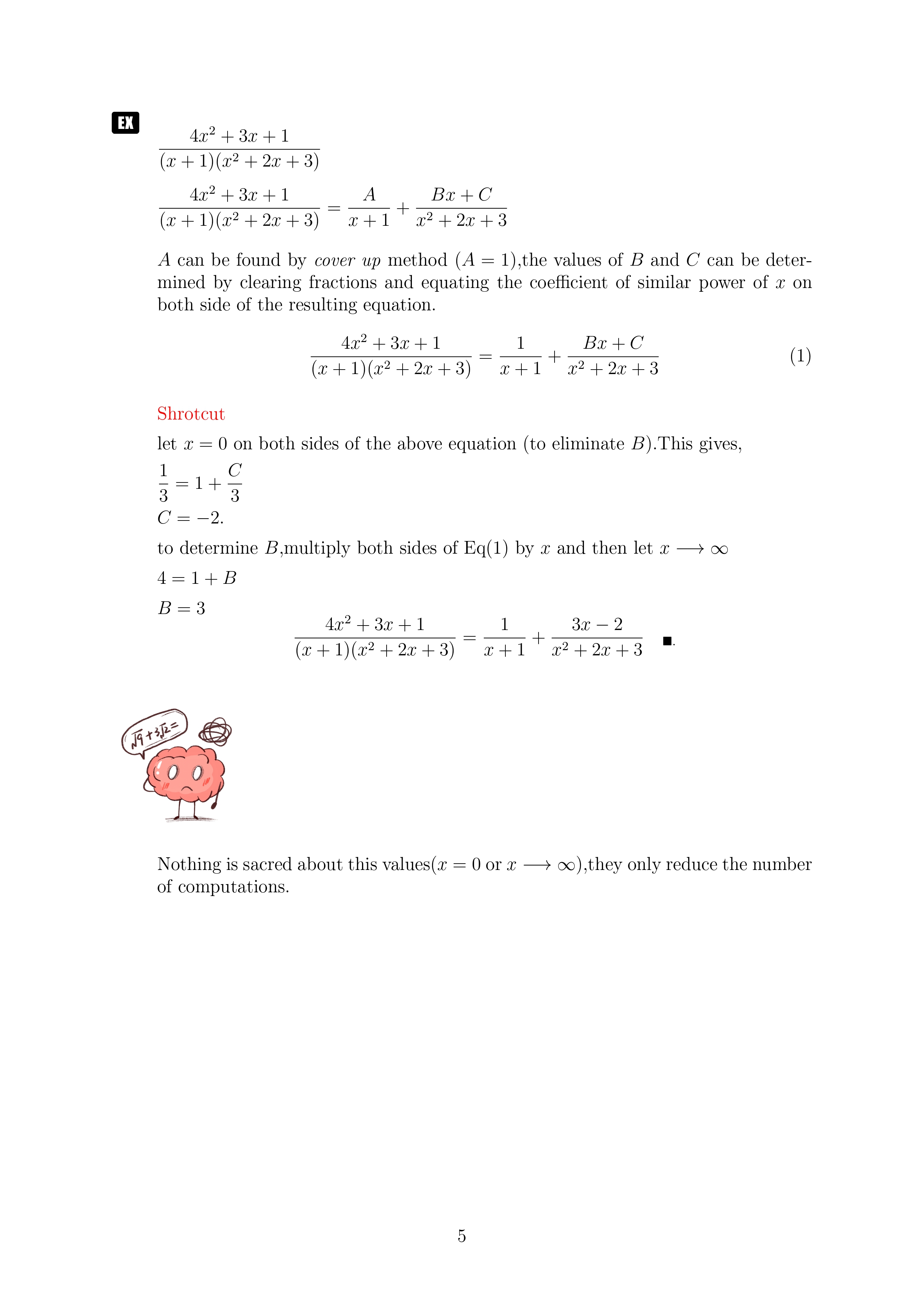
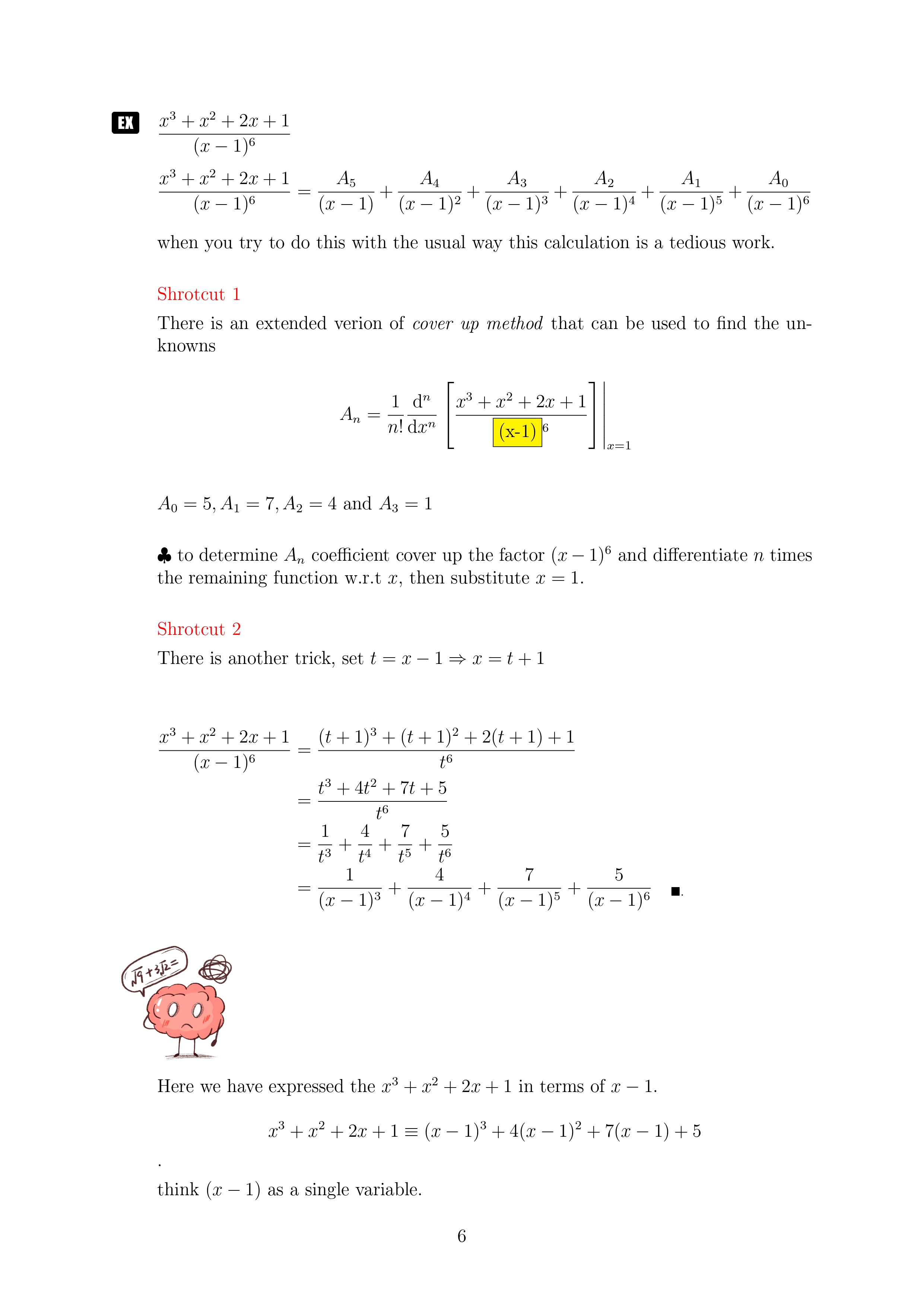
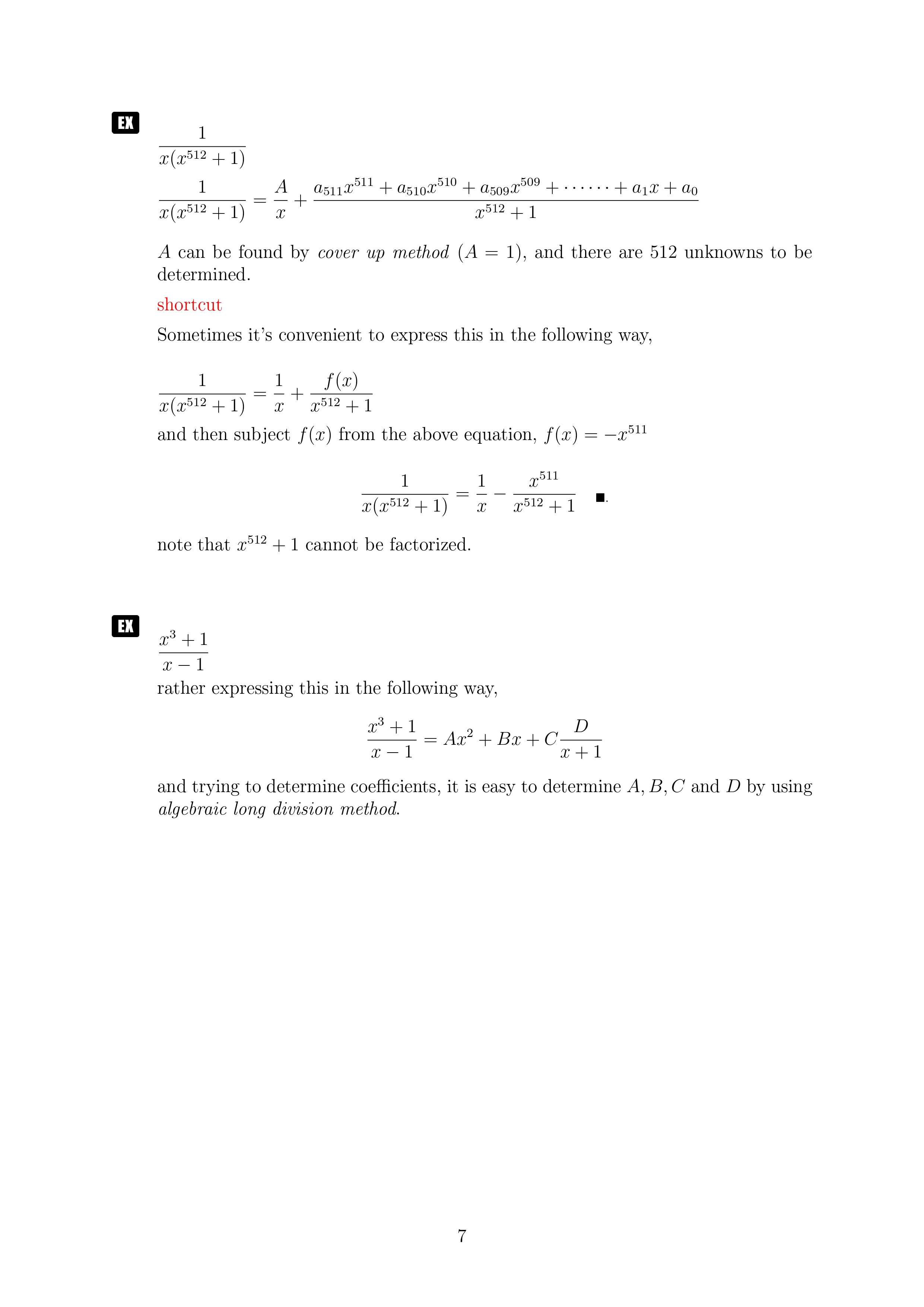
See lessPartial fraction






See lessIllustrating powers of sums by geometrical means
Sum of squares This visual proof by Martin Gardner
Sum of squares
This visual proof by Martin Gardner
See lessIllustrating powers of sums by geometrical means
Sum of integers This visual proof by Ian Richards
Sum of integers
This visual proof by Ian Richards
See lessFunctions
For a function f : A→B, the set A is called the domain of f . (Think of the domain as the set of possible “input values” for f .) The set B is called the codomain of f .(Think of the codomain as a sort of “target” for the outputs.) The range of f is the set { f(a) : a ∈ A} ( Think of the range as thRead more
For a function f : A→B, the set A is called the domain
of f . (Think of the domain as the set of possible “input values” for f .)
The set B is called the codomain of f .(Think of the codomain as a sort of “target” for the outputs.)
The range of f is the set { f(a) : a ∈ A} ( Think of the range as the set of all possible “output values” for f .)
I use the sport of archery to understand this concept. You can think of an arrow as an input value (an arrow can hit only one place on the target board which is also similar to a function having only one relationship for each input value. Finally satisfying one condition to be a function) and the bow as the function. The target board is the codomain and the places where the arrows hit on the target board are called range. Here the target board cannot be set up by the competitor because it is already set by the organizers.
Consider the function f : Z→N, where f (n)= |n|+5.
The domain is Z and the codomain is N.
The range of this function is the set { f(a) : a ∈ Z}={ |n|+5 : a ∈ Z}={5,6,7,…}
Notice that the range is a subset of the codomain N, but it does not (in this case) equal the codomain.
In general, the range of a function is a subset of the codomain. In this sense, the codomain could have been any set that contains the range.
We might just as well have said that this f is a function f : Z→Z, or even f : Z→R.
♣ This illustrates an important point: the codomain of a function is not an intrinsic feature of the function; it is more a matter of choice or context.
In general, the codomain of a function can be any set that contains the function’s range as a subset.
See lessGeometrical approach in combinatorics
See page 375 in the Attachment.
See page 375 in the Attachment.
See lessIndices
As given above this problem can be solved using the look-for-pattern strategy, but the guess and check strategy can also be implemented to solve the same problem. Suppose 𝑥 is the last digit of 2^2015, As 2^2015 is an even number, then 𝑥 =0,2,4,6,8. Consider the number “ 2^2015 – 𝑥 ”, which has 0 (zRead more
As given above this problem can be solved using the look-for-pattern strategy, but the guess and check strategy can also be implemented to solve the same problem.
Suppose 𝑥 is the last digit of 2^2015, As 2^2015 is an even number, then 𝑥 =0,2,4,6,8. Consider the number “ 2^2015 – 𝑥 ”, which has 0 (zero) in its unit place. Alternatively, we will check here which 𝑥 value gives 10|( 2^2015 – 𝑥).
Next, we check whether the following numbers are divisible by 10.
2^2015−0, 2^2015−2, 2^2015−4, 2^2015−6, 2^2015−8.
Among these five numbers, only one number is divisible by 10. ( 2^2015 has only one unique digit in its unit place). All the above numbers are divisible by 2.
Next, check which numbers are divisible by 5.
we know that 𝑥+𝑎|𝑥^n+𝑎^n for any odd integer 𝑛. If we carefully observe and choose values for 𝑥=4,𝑎=1, and 𝑛=1007 (odd).
4+1|4^1007 +1
4+1|2^2014 +1
⇒ 5|2(2^2014 +1)
5|2^2015 +2.
We can use this to check which numbers going to be divisible by 5.
As we know 5 | 2^ 2015 +2, within every five units from that number, is divisible by 5. So here if we move 5 units back from 2^ 2015 +2 we come across 2^ 2015 −3. In the same way, if we go 5 units back we will end up in 2^ 2015 −8. Which means 5|2^ 2015 −8.
2 and 5 are relatively prime numbers, therefore 10|2^ 2015 −8.
Among these numbers 2^ 2015, 2^ 2015−2, 2^ 2015−4, 2^ 2015−6, 2^ 2015−8 only 2^ 2015 −8 is divisible by 10. therefore 8 is the last digit of 2^ 2015. QED
See less